Car Physics and Newton’s Laws of Motion
Isaac Newton is perhaps the greatest scientist that ever lived. The publication of his Philosophiae Naturalis Principia Mathematica (Mathematical Principles of Natural Philosophy) – also known as the Principia – in 1687 revolutionized what we now know as Physics.
His work outlined many of the ‘rules’ of the universe, particularly relating to the way that objects and forces interact with one another. To explain his ideas, Newton had to develop Calculus – demonstrating the radical nature of his theories.
Perhaps most famously in Principia were Newton’s three laws of motion, stating universal rules on how forces operate on objects.
Considering the danger associated with such rules, it’s best to understand what this means when it comes time to get into a moving vehicle. You may think that you only need to understand deductibles and insurance points, but there is much more to safe driving than just keeping a clean record.

Newton was able to apply mathematical principles to seemingly random collisions and other interactions between objects. In doing so, he showed how even the natural world conformed to universal, unchanging, mathematical principles.
By showing that a stone thrown by a small boy is subject to the same calculable forces as the movement of the planets, he created a belief that the universe was understandable through examination, ushering in an age of empiricism and rationalism that culminated in the Scientific Revolution and the Enlightenment.

Because Newton’s work is based on universal applications, it has applications that Newton himself could never have conceived of. One of the most important of these is car technology.
Cars are fundamentally rooted in the principle of moving objects with large masses, requiring the exertion of large forces. Car accidents and collisions are based on opposing forces being exerted, often over very short periods of time.
Understanding Newton’s forces can help with mitigating the damage of these forces to both car and passenger.
What are the key points of car physics?
The first of the key points of car physics, or vehicle physics, is that driving a car produces kinetic energy. When it comes to vehicles of unequal size colliding, the bigger vehicle will have slower deceleration. Upon hitting an
object, the vehicle can move in the opposite direction.
Who is famous for having introduced the three laws of motion?
When identifying who is famous for having introduced the three laws of motion, few names usually come to mind. Sir Isaac Newton is the person behind the three laws of motion, which is applied to car physics today.
Within his pages of Principia, Newton also displayed his law of gravity as a case study of his laws of motion. All matter exerts a force that pulls all other matter towards its center. The strength of the force depends on the mass of the object: the Sun has more gravity than Earth, which in turn has more gravity than an apple.
Newton’s laws of motion and theory of gravity described Earth’s annual journey around the Sun. Earth would move straight forward through the universe, but the Sun uses a constant pull on our planet. This force bends Earth’s path toward the Sun, pulling the planet into an elliptical orbit (almost circular) orbit. His physical theories also explain and predict the tides.
What is the first law of motion?
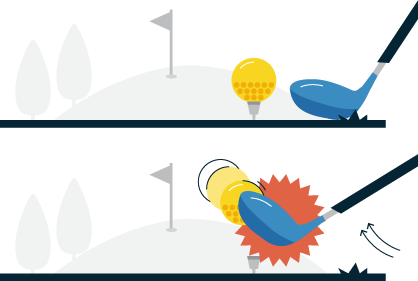
Newton’s first law of motion states that every object will remain at either rest or in uniform motion in a straight line until an external force acts upon it. This is what is known as inertia.
What this means in practice is that if there is no net force acting upon an object at rest (i.e. if all the forces acting on an object cancel one another out) the object will continue to move in a constant velocity – including if the velocity is zero. A change in the net force on an object will cause the velocity of the object to change.
In layman’s terms

When you bowl a bowling ball down the lane (a slick surface with little friction) the bowling ball will continue at the same velocity until it hits the pins.
Because there are no other forces acting on the bowling ball, it will continue at the same velocity as you released it, not slowing down until it experiences a force that cancels out its velocity (i.e. when it hits the pins or the end of the lane).
What is the second law of motion?
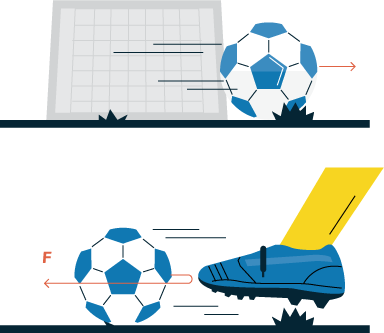
The second law of motion relates to how an object’s behavior changes when surrounding forces are not balanced. According to this law, an object’s acceleration depends upon two things: the force applied to that object and the object’s mass.
Increasing the force on the object directly increases the object’s acceleration and increasing the object’s mass inversely decreases the object’s acceleration.
The force acting on an object is equal to the object’s mass multiplied by its acceleration.
This is described with the equation:
F = m x a
In layman’s terms

The larger the mass of an object, the more force required to get it to move at a certain speed. For example, in football when a quarterback throws a football, they are applying a certain amount of force to it. As they do this, the football accelerates as a direct response to that force.
If a quarterback throws an object with a larger mass, such as a rock, they will need to apply a much greater amount of force in order to get it to have the same amount of acceleration.
What is the third law of motion?

The third law is perhaps the most famous; it states that for every action, there is an equal and opposite reaction.
If one object exerts a force on another, the second object will exert an equal force (in the opposite direction) on the first object.
In layman’s terms

Imagine you are standing on a slick, icy surface and you attempt to give a single, powerful push to a shopping cart (or another relatively heavy object).
You may succeed in moving the cart forwards, but you are likely to also push yourself backward. This is an example of the equal and opposite principle. As you push on the cart, the force you exert will be matched by the cart, thus sending you backward.
How does the conservation of energy work?
An idea connected with Newton’s three laws of motion – although not explicitly stated until the nineteenth century – was the principle of the conservation of energy.
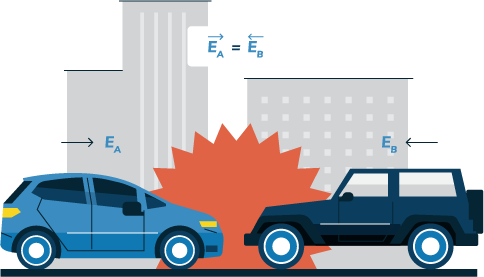
In 1842, Julius Robert von Mayer (and, independently, in 1843, James Prescott Joule) wrote that energy can neither be created or destroyed and that all forces with an isolated system must equal one another.
Put simply, in a vacuum (i.e. with no other forces acting on an object) an object moving in a straight line with a force of x can only be stopped by the application of a force equal to x in the opposite direction. All forces in this equation equal one another and energy has neither been created nor destroyed.

What this means in practice is that concepts such as potential energy (gravitational and elastic), kinetic energy, and heat and light all play a role in ‘balancing’ out forces. This is important for calculating the effect of forces on cars and passengers and is an integral part of understanding Newton’s three laws.
What are the effects on passengers?
Seat belts
When you are driving in a car, you have huge amounts of kinetic energy, because you are moving through space at a relatively high speed. In the case of a collision, where the stopping distance is relatively short, the impact force on a body is extremely high.
What causes a large number of injuries when it comes to collisions is the sudden deceleration caused by stopping. This exerts huge forces on the body, often leading to internal trauma.

In designing safer cars, one of the biggest issues faced by manufacturers is the need to stop a passenger in the case of a collision, without stopping them so quickly as to cause injury.

If a passenger were to not wear a seat belt, they would not stop when the vehicle did, causing them to continue to move in the same direction until an equal and opposite force acts upon them.
This usually amounts to slamming into the steering wheel or dashboard or hitting the windshield; if they are in the rear seats, it leads to them exerting huge forces on the seats in front of them, which can be deadly for those sitting in the front seats.

The invention of the three-point seat belt provided a means by which the stopping of the car would lead to the stopping of the passenger. In effect, the seat belt exerts a force opposite and equal to the force of the kinetic energy of the passenger, stopping them from moving forward.

However, because of the sudden, large force being exerted on a small area, there is the potential for the seat belt to itself cause injury (although this is far, far less of a danger than not wearing a seat belt).

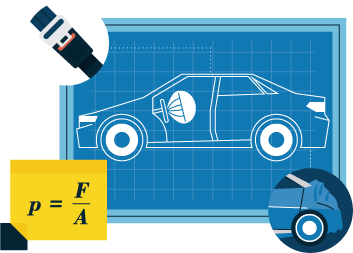
The use of an air bag aids in this respect because, while not diminishing the overall force on the body, it exerts equal pressure on all points coming into contact with it. Famed physicist Blaise Pascal’s principle holds that: Pressure = force/area.
p = F / A
Because the air bag maximizes the area that a person comes into contact with, the pressure is diminished, and therefore the force of sudden deceleration is less likely to cause injury.
Ultimately, therefore, the combination of a seat belt and air bag is optimal because they exert the forces required to stop a passenger, while also dispersing the forces to minimize injuries from deceleration.
Mass of cars
Because of Newton’s Second Law, and the idea that force is equal to mass multiplied by acceleration, the larger a vehicle, the more likely it is to ‘win’ a collision with another vehicle.

Consider a head-on collision between a vehicle with a mass of 2000 kilograms and one with a mass of 1000 kilograms.
Assuming they are both traveling at the same speed – albeit in opposing directions – the larger vehicle has twice the force of the smaller ones.
This means that the head-on collision will cause the smaller vehicle to be pushed backward, or for the larger vehicle to drive over the smaller one.
The larger vehicle will, therefore, experience a slower deceleration. The smaller vehicle will not only decelerate extremely rapidly, but the larger vehicle may actually crush it (depending on the relative axle height).

This demonstrates why the mass of a vehicle is inversely proportional to the fatality of crashes, as demonstrated by the graph below. As a vehicle gets heavier, it is less likely to collide with a heavier vehicle, meaning that it is less likely to ‘lose’ a collision.
Assessment of Driver Fatalities in Single Car Accidents as a Function of Vehicle Mass
- 16-24 yrs
- 25-34 yrs
- >35 yrs
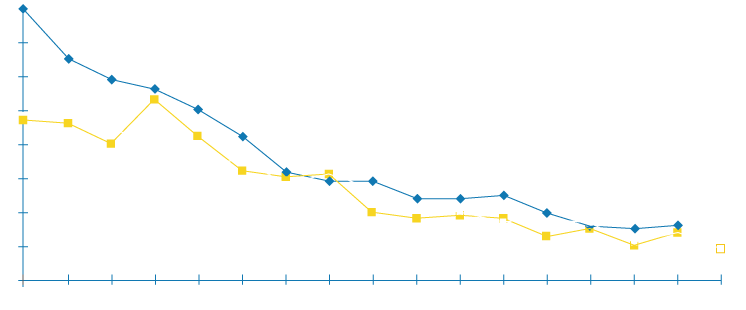
Driver Fatalities / Pedestrian Fatalities
Vehicle Mass in Kilograms
Data from “The Science of Traffic Safety” by Leonard Evans
There are mitigating factors in this, however, as vehicles are increasingly being designed so that collisions are less dependent on weight.
Furthermore, with more modern materials used in cars, overall vehicle mass has diminished. However, according to Newton’s principles, the general rule of thumb is that a larger vehicle is more likely to ‘win’ a collision, resulting in fewer injuries for the passengers in that vehicle.
Crumple Zones
Crumple zones in cars are designed with fundamental Newtonian principles in mind. One of the biggest problems, when a car collides with another object, is the capacity of a car to rebound (i.e. to hit an object, decelerate to a velocity of zero, and then accelerate in the opposite direction). Imagine a car hitting a wall and then bouncing off.

This exerts huge amounts of force on the occupants of the car. In the 1960s and 1970s car manufacturers began using Newtonian principles to determine that it was optimal for a car to crumple rather than remain rigid in the face of a collision.
Although a crumpled car looks like it has experienced more damage, it has, in fact, used Newtonian principles to diminish force.
An additional concept here is ‘impulse,’ which is a measure of how much the momentum changes as a result of a force acting on it for a period of time. The formula for impulse is the net force multiplied by the time that force is applied.

I = F x t
Since impulse equals the change in momentum, an object with 50 units of momentum needs 50 units of impulse to stop it. In a collision, the impulse will always remain the same. However, increasing the time of a collision directly diminishes the average force.
A crumple zone actually extends the time of a collision, meaning the car takes longer to stop. This decreases the force by a proportional amount, thereby lowering the potential for injury.

For example, if the time taken to stop is extended by a factor of 100 with the use of a crumple zone, the force is reduced by a factor of 100, meaning that the passengers have less of a change in momentum.
This has major ramifications for the safety of the passengers, and crumple zones are now standard on vehicles.
Ultimately, therefore, Newton’s work is relevant to the study of car safety because of the universality (and indeed the relative simplicity) of his principles.
Understanding how and why forces interact allows for car manufacturers to envision ways to mitigate their effects. A key example of this is crumple zones on cars. In effect, this is a way to increase the time it takes for a car to stop in the case of a collision, thereby reducing the negative effect of the forces on the human body.
A testament to the success and importance of Newton’s ideas is the extent to which they now seem so simple. Hence why the work of a seventeenth-century English mathematician has ramifications for the design of air bags, crumple zones, and other innovative pieces of car safety.
Are there sources for further reading?
- https://ef.engr.utk.edu/hyperphysics/hbase/seatb2.html
- https://www.physicsclassroom.com/class/momentum/Lesson-1/Real-World-Applications
- http://web.physics.ucsb.edu/~lecturedemonstrations/Composer/Pages/36.27.html
- https://www4.uwsp.edu/physastr/hinaus/Phys203/crumple_zone_lab.htm
